Tamilnadu state board 10th Maths answers Chapter 1 Relations and Functions Exercise 1.2 - New syllabus
10th maths Ex 1.2
- Question 1.
(i) R₁ = {(2, 1), (7, 1)}
(ii) R₂ = {(-1, 1)}
(iii) R₃ = {(2, -1), (7, 7), (1, 3)}
(iv) R₄ = {(7,-1), (0, 3), (3, 3), (0, 7)}
(i) A = {1, 2, 3, 7}, B = {3, 0,-1, 7}
- Solution:
It is not a relation there is no element as 1 in B.
(ii) R₂ = {(-1, 1)}
It is not [∵ -1 ∉ A, 1 ∉ B]
(iii) R₃ = {(2, -1), (7, 7), (1, 3)}
It is a relation.
R₄ = {(7,-1), (0, 3), (3, 3), (0, 7)}
It is also not a relation. [∵ 0 ∉ A]
- Question 2.
Let A = {1, 2, 3, 4, ….., 45} and R be the relation defined as “is square of ” on A. Write R as a subset of A × A. Also, find the domain and range of R.- Answer:
The relation is defined as “is square of’
R = {(1,1) (2, 4) (3, 9)
(4, 16) (5,25) (6, 36)}
Domain of R = {1, 2, 3, 4, 5, 6}
Range of R = {1, 4, 9, 16, 25, 36}
- Question 3.
A Relation R is given by the set {(x, y) /y = x + 3, x ∈ {0, 1, 2, 3, 4, 5}}. Determine its domain and range.Solution:
x = {0, 1, 2, 3, 4, 5}
y = x + 3
⇒ y = {3, 4, 5, 6, 7, 8}
R = {(x, y)}
= {(0, 3),(1, 4),(2, 5),(3, 6), (4, 7), (5, 8)}
Domain of R = {0, 1, 2, 3, 4, 5}
Range of R = {3, 4, 5, 6, 7, 8}
- Question 4.
Represent each of the given relation by (a) an arrow diagram, (b) a graph and (c) a set in roster form, wherever possible.(i) {(x, y)|x = 2y,x ∈ {2, 3, 4, 5},y ∈ {1, 2, 3, 4)
(ii) {(x, y)|y = x + 3, x, y are natural numbers <10}
Solution:
(i){(x, y)|x = 2y, x ∈ {2, 3, 4, 5},y ∈ {1, 2, 3, 4}} R = (x = 2y)2 = 2 × 1 = 2
4 = 2 × 2 = 4
(c) {(2, 1), (4, 2)}
(ii) {(x, y)|y = x + 3, x,+ are natural numbers <10}
x = {1, 2, 3, 4, 5, 6, 7, 8, 9} R = (y = x + 3)
y = {1, 2, 3, 4, 5, 6, 7, 8, 9}
R = {(1, 4), (2, 5), (3, 6), (4, 7), (5, 8), (6, 9)}
- Question 5.
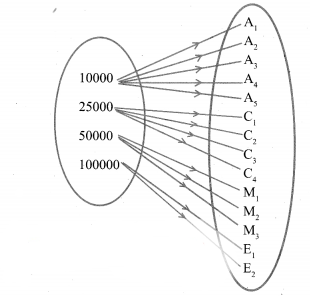
A company has four categories of employees given by Assistants (A), Clerks (C), Managers (M) and an Executive Officer (E). The company provide ₹10,000, ₹25,000, ₹50,000 and ₹1,00,000 as salaries to the people who work in the categories A, C, M and E respectively. If A, A₂, A₃, A₄ and As were Assistants; C₁, C₂, C₃, C4 were Clerks; M₁, M₂, M₃ were managers and E₁,E₂ were Executive officers and if the relation R is defined by xRy, where x is the salary given to person y, express the relation R through an ordered pair and an arrow diagram.
- Solution:
A – Assistants → A₁, A₂, A₃, A4₄, A₅
C – Clerks → C₁, C2₂, C₃, C4₄
D – Managers → M₁, M₂, M₃
E – Executive officer → E₁, E₂
(a) R = {(10,000, A₁), (10,000, A₂), (10,000, A₃),
(10,000, A₄), (10,000, A₅), (25,000, C₁),
(25,000, C₂), (25,000, C₃), (25,000, C₄),
(50,000, M₁), (50,000, M₂), (50,000, M₃),
(1,00,000, E₁), (1,00,000, E₂)}

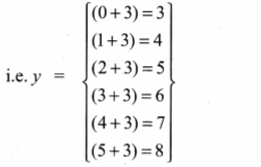



0 Comments:
Post a Comment